Theoretical Excellence

Although Aristotle in general had a more empirical and experimental attitude than Plato, modern science did not come into its own until Plato's Pythagorean confidence in the mathematical nature of the world returned with Kepler, Galileo, and Newton. For instance, Aristotle, relying on a theory of opposites that is now only of historical interest, rejected Plato's attempt to match the Platonic Solids with the elements -- while Plato's expectations are realized in mineralogy and crystallography, where the Platonic Solids occur naturally.Plato and Aristotle, Up and Down-Kelley L. Ross, Ph.D.

This is the first introduction then that is very important to me about what is perceived as a mathematical framework. So it is not such an effort to think about our world and think hmmmm.... a mathematical abstract of our reality is there to be discovered. I first noticed this attribute in Pascal's triangle.

Nineteenth Century Geometry by Roberto Torretti

The sudden shrinking of Euclidean geometry to a subspecies of the vast family of mathematical theories of space shattered some illusions and prompted important changes in our the philosophical conception of human knowledge. Thus, for instance, after these nineteenth-century developments, philosophers who dream of a completely certain knowledge of right and wrong secured by logical inference from self-evident principles can no longer propose Euclidean geometry as an instance in which a similar goal has proved attainable. The present article reviews the aspects of nineteenth century geometry that are of major interest for philosophy and hints in passing, at their philosophical significance.

While I looked further into the world of Pythagorean developments I wondered how such an abstract could have ever lead to the world of non-euclidean geometries. There is this progression of the geometries that needed to be understood. It included so many people that we only now acknowledge the greatest names but it is in the exploration of "theoretical excellence" that we gain access to the spirituality's of the mathematical world.

"I’m a Platonist — a follower of Plato — who believes that one didn’t invent these sorts of things, that one discovers them. In a sense, all these mathematical facts are right there waiting to be discovered."Donald (H. S. M.) Coxeter

While some would wonder what value this exploration into such mathematical abstracts, how could we describe for ourselves the ways things would appear at such levels microscopically reduced, has an elemental quality to it? Yes, I have gone to one extreme, and understand, it included so many different mathematics, how could we ever understand this effort and assign it's rightful place in history? Theoretics then, is this effort?

How Strange the elements of our world?

The crystalline state is the simplest known example of a quantum , a stable state of matter whose generic low-energy properties are determined by a higher organizing principle and nothing else. Robert Laughlin

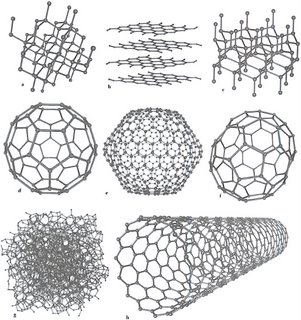
This illustration depicts eight of the allotropes (different molecular configurations) that pure carbon can take:

a) Diamond
b) Graphite
c) Lonsdaleite
d) Buckminsterfullerene (C60)
e) C540
f) C70
g) Amorphous carbon
h) single-walled carbon nanotube

Review of experiments

Graphite exhibits elastic behaviour and even improves its mechanical strength up to the temperature of about 2500 K. Measured changes in ultrasonic velocity in graphite after high temperature creep shows marked plasticity at temperatures above 2200 K [16]. From the standpoint of thermodynamics, melting is a phase transition of the first kind, with an abrupt enthalpy change constituting the heat of melting. Therefore, any experimental proof of melting is associated with direct recording of the temperature dependence of enthalpy in the neighbourhood of a melting point. Pulsed heating of carbon materials was studied experimentally by transient electrical resistance and arc discharge techniques, in millisecond and microsecond time regime (see, e.g., [17, 18]), and by pulsed laser heating, in microsecond, nanosecond and picosecond time regime (see, e.g., [11, 19, 20]). Both kind of experiments recorded significant changes in the material properties (density, electrical and thermal conductivity, reflectivity, etc. ) within the range 4000-5000 K, interpreted as a phase change to a liquid state. The results of graphite irradiation by lasers suggest [11] that there is at least a small range of temperatures for which liquid carbon can exist at pressure as low as 0.01 GPa. The phase boundaries between graphite and liquid were investigated experimentally and defined fairly well.