Nature is the Architect

.....and we are it's builders?



So beyond indeed, is the static realization of the structure of things. This is a more definable recognition of something that is very fluid and expressive. It is by our own humanistic natures that we like to compartmentalize?

"There comes a time when the mind takes a higher plane of knowledge but can never prove how it got there. All great discoveries have involved such a leap. The important thing is not to stop questioning." Albert Einstein (1879- 1955)

While this quote of Einstein is somewhat revealing of what can flash across the mind, it is by intense work that such a time allows for things to gather, and in this work, it will inevitable makes sense. Such cultivation allows for new things to be born and in such nurture and contemplation, something will eventually emerge.

A picture of flux lines in QED (left) and QCD (right).

Although it didn't properly describe strong interactions, in studying string theory physicists stumbled upon an amazing mathematical structure. String theory has turned out to be far richer than people originally anticipated. For example, people found that a certain vibrational state of the string has zero mass and spin 2. According to Einstein's theory of gravity, the gravitational force is mediated by a particle with zero mass and spin 2. So string theory is, among many other things, a theory of gravity!

See: Why Strings

This points to a reductionistic view about the nature of reality. That we are part and parcel creating the constituents of the reality that we see, has a glue that binds, and keeps it together. For each this glue is a process that has meaning for each of us. While one would wonder where such motivation would allow each to perceive it as so one might ask what value is assign each stage of expression to see that such a scale has been reduce to a quality of a kind? It's music?


Cover of Hiding in the Mirror: The Mysterious Allure of Extra Dimensions, from Plato to String Theory and Beyond by Lawrence M. Krauss
Viking Press

Guide Review - Hiding in the Mirror by Lawrence Krauss
In Hiding in the Mirror, astrophysicist and cosmologist Lawrence M. Krauss addresses the concept of extra dimensions, from its appearance in popular culture such as Alice in Wonderland and The Time Machine to theoretical physics areas such as the theory of relativity and string theory. In fact, I would say that the book splits roughly 50/50 between cultural and scientific topics, which is part of the point of the book (that extra dimensions are tied to both areas), but for those who are specifically interested in the scientific aspects there are other books (such as Lisa Randall's Warped Passages) which address the scientific aspects in far more depth.

According to Krauss, extra dimensions have captured the human imagination well before it entered into exploration by physics in the last century or so. The book covers how the concepts were viewed by those in the past, as well as more recent science fiction, such as Star Trek (one of Krauss' favorite topics, as author of the bestselling The Physics of Star Trek). Much of this material is entertaining, but for those who are wanting to get to the heart of the physics, it can feel like filler.

About 100 pages of the book focuses on the recent work to find a unified theory of quantum gravity, focusing predominantly on string theory (with some mention of predecessors). This has been one of the areas where extra dimensions have become extremely dominant. Though Krauss exhibits some genuine skepticism about the track string theory is on, I think calling the book a criticism of string theory would be going a bit far. Krauss is placing string theory within a larger framework of extra dimensional movements in the past, many of which have proved incredibly enlightening and some of which have not done much. It's left to other books to determine whether string theory has any scientific merit.

See:Book Review: Hiding in the Mirror

***

See Also:

Where are my keys?

So string theory is, among many other things, a theory of gravity!

EOT-WASH GROUP(4)

Who said it?

At this point in the development, although geometry provided a common framework for all the forces, there was still no way to complete the unification by combining quantum theory and general relativity. Since quantum theory deals with the very small and general relativity with the very large, many physicists feel that, for all practical purposes, there is no need to attempt such an ultimate unification. Others however disagree, arguing that physicists should never give up on this ultimate search, and for these the hunt for this final unification is the ‘holy grail’. Michael Atiyah

"No Royal Road to Geometry?"

Click on the Picture

Are you an observant person? Look at the above picture. Why ask such a question as to, "No Royal Road to Geometry?" This presupposes that a logic is formulated that leads not only one by the "phenomenological values" but by the very principal of logic itself.

All those who have written histories bring to this point their account of the development of this science. Not long after these men came Euclid, who brought together the Elements, systematizing many of the theorems of Eudoxus, perfecting many of those of Theatetus, and putting in irrefutable demonstrable form propositions that had been rather loosely established by his predecessors. He lived in the time of Ptolemy the First, for Archimedes, who lived after the time of the first Ptolemy, mentions Euclid. It is also reported that Ptolemy once asked Euclid if there was not a shorter road to geometry that through the Elements, and Euclid replied that there was no royal road to geometry. He was therefore later than Plato's group but earlier than Eratosthenes and Archimedes, for these two men were contemporaries, as Eratosthenes somewhere says. Euclid belonged to the persuasion of Plato and was at home in this philosophy; and this is why he thought the goal of the Elements as a whole to be the construction of the so-called Platonic figures. (Proclus, ed. Friedlein, p. 68, tr. Morrow)

I don't think I could of made it any easier for one, but to reveal the answer in the quote. Now you must remember how the logic is introduced here, and what came before Euclid. The postulates are self evident in his analysis but, little did he know that there would be a "Royal Road indeed" to geometry that was much more complex and beautiful then the dry implication logic would reveal of itself.

It's done for a reason and all the geometries had to be leading in this progressive view to demonstrate that a "projective geometry" is the final destination, although, still evolving?

Eventually it was discovered that the parallel postulate is logically independent of the other postulates, and you get a perfectly consistent system even if you assume that parallel postulate is false. This means that it is possible to assign meanings to the terms "point" and "line" in such a way that they satisfy the first four postulates but not the parallel postulate. These are called non-Euclidean geometries. Projective geometry is not really a typical non-Euclidean geometry, but it can still be treated as such.

In this axiomatic approach, projective geometry means any collection of things called "points" and things called "lines" that obey the same first four basic properties that points and lines in a familiar flat plane do, but which, instead of the parallel postulate, satisfy the following opposite property instead:

The projective axiom: Any two lines intersect (in exactly one point).

If you are "ever the artist" it is good to know in which direction you will use the sun, in order to demonstrate the shadowing that will go on into your picture. While you might of thought there was everything to know about Plato's cave and it's implication I am telling you indeed that the logic is a formative apparatus concealed in the geometries that are used to explain such questions about, "the shape of space."

The Material World

There are two reasons that having mapped E8 is so important. The practical one is that E8 has major applications: mathematical analysis of the most recent versions of string theory and supergravity theories all keep revealing structure based on E8. E8 seems to be part of the structure of our universe.

The other reason is just that the complete mapping of E8 is the largest mathematical structure ever mapped out in full detail by human beings. It takes 60 gigabytes to store the map of E8. If you were to write it out on paper in 6-point print (that's really small print), you'd need a piece of paper bigger than the island of Manhattan. This thing is huge.

Polytopes and allotrope are examples to me of "shapes in their formative compulsions" that while very very small in their continuing expression, "below planck length" in our analysis of the world, has an "formative structure" in the case of the allotrope in the material world. The polytopes, as an abstract structure of math thinking about the world. As if in nature's other ways.



This illustration depicts eight of the allotropes (different molecular configurations) that pure carbon can take:

a) Diamond
b) Graphite
c) Lonsdaleite
d) Buckminsterfullerene (C60)
e) C540
f) C70
g) Amorphous carbon
h) single-walled carbon nanotube

Review of experiments

Graphite exhibits elastic behaviour and even improves its mechanical strength up to the temperature of about 2500 K. Measured changes in ultrasonic velocity in graphite after high temperature creep shows marked plasticity at temperatures above 2200 K [16]. From the standpoint of thermodynamics, melting is a phase transition of the first kind, with an abrupt enthalpy change constituting the heat of melting. Therefore, any experimental proof of melting is associated with direct recording of the temperature dependence of enthalpy in the neighbourhood of a melting point. Pulsed heating of carbon materials was studied experimentally by transient electrical resistance and arc discharge techniques, in millisecond and microsecond time regime (see, e.g., [17, 18]), and by pulsed laser heating, in microsecond, nanosecond and picosecond time regime (see, e.g., [11, 19, 20]). Both kind of experiments recorded significant changes in the material properties (density, electrical and thermal conductivity, reflectivity, etc. ) within the range 4000-5000 K, interpreted as a phase change to a liquid state. The results of graphite irradiation by lasers suggest [11] that there is at least a small range of temperatures for which liquid carbon can exist at pressure as low as 0.01 GPa. The phase boundaries between graphite and liquid were investigated experimentally and defined fairly well.

Sean Carroll:But if you peer closely, you will see that the bottom one is the lopsided one — the overall contrast (representing temperature fluctuations) is a bit higher on the left than on the right, while in the untilted image at the top they are (statistically) equal. (The lower image exaggerates the claimed effect in the real universe by a factor of two, just to make it easier to see by eye.)

See The Lopsided Universe-.

#36.Plato on Jun 12th, 2008 at 10:17 am

Lawrence,

Thanks again.

“I’m a Platonist — a follower of Plato — who believes that one didn’t invent these sorts of things, that one discovers them. In a sense, all these mathematical facts are right there waiting to be discovered.”Harold Scott Macdonald (H. S. M.) Coxeter

Moving to polytopes or allotrope seem to have values in science? Buckminister Fuller and Richard Smalley in terms of allotrope.

I was looking at Sylvestor surfaces and the Clebsch diagram. Cayley too. These configurations to me were about “surfaces,” and if we were to allot a progression to the “projective geometries” here in relation to higher dimensional thinking, “as the polytope[E8]“(where Coxeter[I meant to apologize for misspelling earlier] drew us to abstraction to the see “higher dimensional relations” toward Plato’s light.)

As the furthest extent of the Conjecture , how shall we place the dynamics of Sylvestor surfaces and B Fields in relation to the timeline of these geometries? Historically this would seem in order, but under the advancement of thinking in theoretics does it serve a purpose? Going beyond “planck length” what is a person to do?

Thanks for the clarifications on Lagrange points. This is how I see the WMAP.

Diagram of the Lagrange Point gravitational forces associated with the Sun-Earth system. WMAP orbits around L2, which is about 1.5 million km from the Earth. Lagrange Points are positions in space where the gravitational forces of a two body system like the Sun and the Earth produce enhanced regions of attraction and repulsion. The forces at L2 tend to keep WMAP aligned on the Sun-Earth axis, but requires course correction to keep the spacecraft from moving toward or away from the Earth.

Such concentration in the view of Sean’s group of the total WMAP while finding such a concentration would be revealing would it not of this geometrical instance in relation to gravitational gathering or views of the bulk tendency? Another example to show this fascinating elevation to non-euclidean, gravitational lensing, could be seen in this same light.

Such mapping would be important to the context of “seeing in the whole universe.”

See:No Royal Road to Geometry
Allotropes and the Ray of Creation
Pasquale Del Pezzo and E8 Origination?
Projective Geometries

Theoretical Excellence

Although Aristotle in general had a more empirical and experimental attitude than Plato, modern science did not come into its own until Plato's Pythagorean confidence in the mathematical nature of the world returned with Kepler, Galileo, and Newton. For instance, Aristotle, relying on a theory of opposites that is now only of historical interest, rejected Plato's attempt to match the Platonic Solids with the elements -- while Plato's expectations are realized in mineralogy and crystallography, where the Platonic Solids occur naturally.Plato and Aristotle, Up and Down-Kelley L. Ross, Ph.D.

This is the first introduction then that is very important to me about what is perceived as a mathematical framework. So it is not such an effort to think about our world and think hmmmm.... a mathematical abstract of our reality is there to be discovered. I first noticed this attribute in Pascal's triangle.

Nineteenth Century Geometry by Roberto Torretti

The sudden shrinking of Euclidean geometry to a subspecies of the vast family of mathematical theories of space shattered some illusions and prompted important changes in our the philosophical conception of human knowledge. Thus, for instance, after these nineteenth-century developments, philosophers who dream of a completely certain knowledge of right and wrong secured by logical inference from self-evident principles can no longer propose Euclidean geometry as an instance in which a similar goal has proved attainable. The present article reviews the aspects of nineteenth century geometry that are of major interest for philosophy and hints in passing, at their philosophical significance.

While I looked further into the world of Pythagorean developments I wondered how such an abstract could have ever lead to the world of non-euclidean geometries. There is this progression of the geometries that needed to be understood. It included so many people that we only now acknowledge the greatest names but it is in the exploration of "theoretical excellence" that we gain access to the spirituality's of the mathematical world.

"I’m a Platonist — a follower of Plato — who believes that one didn’t invent these sorts of things, that one discovers them. In a sense, all these mathematical facts are right there waiting to be discovered."Donald (H. S. M.) Coxeter

While some would wonder what value this exploration into such mathematical abstracts, how could we describe for ourselves the ways things would appear at such levels microscopically reduced, has an elemental quality to it? Yes, I have gone to one extreme, and understand, it included so many different mathematics, how could we ever understand this effort and assign it's rightful place in history? Theoretics then, is this effort?

How Strange the elements of our world?

The crystalline state is the simplest known example of a quantum , a stable state of matter whose generic low-energy properties are determined by a higher organizing principle and nothing else. Robert Laughlin

This illustration depicts eight of the allotropes (different molecular configurations) that pure carbon can take:

a) Diamond
b) Graphite
c) Lonsdaleite
d) Buckminsterfullerene (C60)
e) C540
f) C70
g) Amorphous carbon
h) single-walled carbon nanotube

Review of experiments

Graphite exhibits elastic behaviour and even improves its mechanical strength up to the temperature of about 2500 K. Measured changes in ultrasonic velocity in graphite after high temperature creep shows marked plasticity at temperatures above 2200 K [16]. From the standpoint of thermodynamics, melting is a phase transition of the first kind, with an abrupt enthalpy change constituting the heat of melting. Therefore, any experimental proof of melting is associated with direct recording of the temperature dependence of enthalpy in the neighbourhood of a melting point. Pulsed heating of carbon materials was studied experimentally by transient electrical resistance and arc discharge techniques, in millisecond and microsecond time regime (see, e.g., [17, 18]), and by pulsed laser heating, in microsecond, nanosecond and picosecond time regime (see, e.g., [11, 19, 20]). Both kind of experiments recorded significant changes in the material properties (density, electrical and thermal conductivity, reflectivity, etc. ) within the range 4000-5000 K, interpreted as a phase change to a liquid state. The results of graphite irradiation by lasers suggest [11] that there is at least a small range of temperatures for which liquid carbon can exist at pressure as low as 0.01 GPa. The phase boundaries between graphite and liquid were investigated experimentally and defined fairly well.

Solidification of Geometrical Presence

While I might infer the "attributes of Coxeter here," it is with the understanding such a dimensional perspective which has it's counterpart in the result of what manifests as matter creations. Yet we have taken our views down to the "powers of ten" to think of what could manifest even before we see the result in nature.

When you go to the site by PBS of where, Nano: Art Meets Science, make sure you click on the lesson plan to the right.



Buckyballs

Visitors' shadows manipulate and reshape projected images of "Buckyballs." "Buckyball," or a buckminsterfullerene molecule, is a closed cage-structure molecule with a carbon network. "Buckyball" was named for R. Buckminster "Bucky" Fuller (1895-1983), a scientist, philosopher and inventor, best known for creating the geodesic dome.
Photo Credit: © 2003 Museum Associates/Los Angeles County Museum

Fundamentally the properties of materials can be changed by nanotechnology. We can arrange molecules in a way that they do not normally occur in nature. The material strength, electronic and optical properties of materials can all be altered using nanotechnology.

See Related information on bucky balls here in this site. This should give some understanding of how I see the greater depth of what manifest in nature, as solids in our world, has some "other" possibilities in dimensional attribute, while it is given association to the mathematical prowess of E8.

I do not know of many who will take in all that I have accumulated in regards to how one may look at their planet, can have the depth of perception that is held in to E8.?

One may say what becomes of the world as it manifest into it's constituent parts, has this energy relation, that it would become all that is in the design of the world around us.



While some scientists puzzle as to the nature of the process of E8, little did they realize that if you move your perception to the way E8 is mapped to 248 dimensions, the image while indeed quite pleasing, you see as a result.

It can include so much information, how would you know that this object of mathematics, is a polytrope of a kind that is given to the picture of science in the geometrical structure of the bucky ball or fullerene.

Allotropes



Diamond and graphite are two allotropes of carbon: pure forms of the same element that differ in structure.

Allotropy (Gr. allos, other, and tropos, manner) is a behaviour exhibited by certain chemical elements: these elements can exist in two or more different forms, known as allotropes of that element. In each different allotrope, the element's atoms are bonded together in a different manner.

For example, the element carbon has two common allotropes: diamond, where the carbon atoms are bonded together in a tetrahedral lattice arrangement, and graphite, where the carbon atoms are bonded together in sheets of a hexagonal lattice.

Note that allotropy refers only to different forms of an element within the same phase or state of matter (i.e. different solid, liquid or gas forms) - the changes of state between solid, liquid and gas in themselves are not considered allotropy. For some elements, allotropes can persist in different phases - for example, the two allotropes of oxygen (dioxygen and ozone), can both exist in the solid, liquid and gaseous states. Conversely, some elements do not maintain distinct allotropes in different phases: for example phosphorus has numerous solid allotropes, which all revert to the same P4 form when melted to the liquid state.

The term "allotrope" was coined by the famous chemist Jöns Jakob Berzelius.

Pasquale Del Pezzo and E8 Origination?

"I’m a Platonist — a follower of Plato — who believes that one didn’t invent these sorts of things, that one discovers them. In a sense, all these mathematical facts are right there waiting to be discovered."Donald (H. S. M.) Coxeter

There are two reasons that having mapped E8 is so important. The practical one is that E8 has major applications: mathematical analysis of the most recent versions of string theory and supergravity theories all keep revealing structure based on E8. E8 seems to be part of the structure of our universe.

The other reason is just that the complete mapping of E8 is the largest mathematical structure ever mapped out in full detail by human beings. It takes 60 gigabytes to store the map of E8. If you were to write it out on paper in 6-point print (that's really small print), you'd need a piece of paper bigger than the island of Manhattan. This thing is huge.

Clifford of Asymptotia drew our attention to this for examination and gives further information and links with which to follow.

He goes on to write,"Let’s not get carried away though. Having more data does not mean that you worked harder to get it. Mapping the human genome project involves a much harder task, but the analogy is still a good one, if not taken too far."

Of course since the particular comment of mine was deleted there, and of course I am okay with that. It did not mean I could not carry on here. It did not mean that I was not speaking directly to the way these values in dimensional perspective were not being considered.

Projective Geometries?

A theorem which is valid for a geometry in this sequence is automatically valid for the ones that follow. The theorems of projective geometry are automatically valid theorems of Euclidean geometry. We say that topological geometry is more abstract than projective geometry which is turn is more abstract than Euclidean geometry.

There had to be a route to follow that would lead one to think in such abstract spaces. Of course, one does not want to be divorced from reality. So one should not think that because the geometry of GR is understood, that you think nothing can come from the microseconds after the universe came into expression.

At this point in the development, although geometry provided a common framework for all the forces, there was still no way to complete the unification by combining quantum theory and general relativity. Since quantum theory deals with the very small and general relativity with the very large, many physicists feel that, for all practical purposes, there is no need to attempt such an ultimate unification. Others however disagree, arguing that physicists should never give up on this ultimate search, and for these the hunt for this final unification is the ‘holy grail’. Michael Atiyah

The Holy Grail sure comes up lots doesn't it:) Without invoking the pseudoscience that Peter Woit spoke of. I thought, if they could use Babar, and Alice then I could use the Holy Grail?

See more info on Coxeter here.

Like Peter I will have to address the "gut feelings" and the way Clifford expressed it. I do not want to practise pseudoscience as Peter is about the landscape.:)



When ones sees the constituent properties of that Gossett polytope 4₂₁ in all it's colours, the complexity of that situation is quite revealing. Might we not think in the time of supergravity, gravity will become weak, in the matter constitutions that form.

As in Neutrino mixing I am asking you to think of the particles as sound as well as think them in relation to the Colour of Gravity. If you were just to see grvaity in it's colourful design and what value that gravity in face of the photon moving within this gravitational field?

We detect the resulting "wah-wah-wah" in properties of the neutrino that appear and disappear. For example, when neutrinos interact with matter they produce specific kinds of other particles.

For example, when neutrinos interact with matter they produce specific kinds of other particles. Catch the neutrino at one moment, and it will interact to produce an electron. A moment later, it might interact to produce a different particle. "Neutrino mixing" describes the original mixture of waves that produces this oscillation effect.

The "geometry of curvature" had to be implied in the outcome, from that quantum world? Yet at it's centre, what is realized? You had to be lead there in terms of particle research to know that you are arriving at the "crossover point." The superfluid does this for examination.

5. Regular polytope: If you keep pulling the hypercube into higher and higher dimensions you get a polytope. Coxeter is famous for his work on regular polytopes. When they involve coordinates made of complex numbers they are called complex polytopes.

Pasquale Del Pezzo, Duke of Cajanello, (1859–1936), was "the most Neapolitan of Neapolitan Mathematicians".

He was born in Berlin (where his father was a representative of the Neapolitan king) on 2 May 1859. He died in Naples on 20 June 1936. His first wife was the Swedish writer Anne Charlotte Leffler, sister of the great mathematician Gösta Mittag-Leffler (1846-1927).

At the University of Naples, he received first a law degree in 1880 and then in 1882 a math degree. He became a pre-eminent professor at that university, teaching Projective Geometry, and remained at that University, as rector, faculty president, etc.

He was mayor of Naples starting in 1919, and he became a senator in the Kingdom of Naples.

His scientific achievements were few, but they reveal a keen ingenuity. He is remembered particularly for first describing what became known as a Del Pezzo surface. He might have become one of the strongest mathematicians of that time, but he was distracted by politics and other interests.

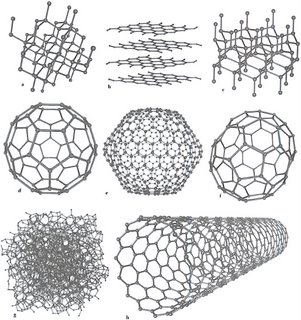
So what chance do we have, if we did not think this geometry was attached to processes that would unfold into the bucky ball or the fullerene of science. To say that the outcome had a point of view that is not popular. I do not count myself as attached to any intelligent design agenda, so I hope people will think I do not care about that.

NATHAN MYHRVOLD

I found the email debate between Smolin and Susskind to be quite interesting. Unfortunately, it mixes several issues. The Anthropic Principle (AP) gets mixed up with their other agendas. Smolin advocates his CNS, and less explicitly loop quantum gravity. Susskind is an advocate of eternal inflation and string theory. These biases are completely natural, but in the process the purported question of the value of the AP gets somewhat lost in the shuffle. I would have liked more discussion of the AP directly

See here for more information

So all the while you see the complexity of that circle and how long it took a computer to map it, it has gravity in it's design, whether we like to think about it or not?

But of course we are talking about the symmetry and any thing less then this would have been assign a matter state, as if symmetrical breaking would have said, this is the direction you are going is what we have of earth?

Isostatic Adjustment is Why Planets are Round?

While one thinks of "rotational values" then indeed one would have to say not any planets is formed in the way the sun does. Yet, in the "time variable understanding" of the earth, we understand why it's shape is not exactly round.



Do you think the earth and moon look round if your were considering Grace?

On the moon what gives us perspective when a crater is formed to see it's geological structure? It's just not a concern of the mining industry, as to what is mined on other orbs, but what the time variable reveals of the orbs structure as well.



Clementine color ratio composite image of Aristarchus Crater on the Moon. This 42 km diameter crater is located on the corner of the Aristarchus plateau, at 24 N, 47 W. Ejecta from the plateau is visible as the blue material at the upper left (northwest), while material excavated from the Oceanus Procellarum area is the reddish color to the lower right (southeast). The colors in this image can be used to ascertain compositional properties of the materials making up the deep strata of these two regions. (Clementine, USGS slide 11)

See more here

IN Search of Mandelstam's Holy Grail

There are two posts that reflect the purpose of this post today. One is Clifford's linked through Lee Smolin's comment and the other, at Backreaction. Good Physics is Conflict

A lot of you may never understand the significance of the mystery that follows the thinking of the Holy Grail. Yet is it more the knowledge that can be gained from all soul's day, that on this occasion we may have called it Halloween.

We celebrated the past, in the living of today? You philosophize, while you become the thoughts of models created by science leaders shared? I do not think any have a "personality disorder" like I do:)

Lee Smolin:

Here is an example of the kind of question I found I needed a book to explore: what to think of the problems that arise from the need for higher dimensions in string theory, such as the problem of moduli stabilization and the vast number of static solutions. To approach this I read books on the early history of GR and unified field theories and learned that higher dimensional compactifications were explored many times between 1914 and 1984 and that close to the beginning these problems were appreciated and discussed by Einstein and others. I weave this story into my book because I find it useful when trying to judge how serious the present issues in string theory are to know how Einstein and many others struggled with the same issues over decades.

So of course when we think of the persons of science who walked before us (shoulders of giants), what are their whole stories, but what is evidenced to us as we read those words? So you compile your data accordingly, and from it, we say at certain spots, how are we to react to the challenge now facing us?



Stanley Mandelstam, Professor Emeritus, Particle Theory

My present research concerns the problem of topology changing in string theory. It is currently believed that one has to sum over all string backgrounds and all topologies in doing the functional integral. I suspect that certain singular string backgrounds may be equivalent to topology changes, and that it is consequently only necessary to sum over string backgrounds. As a start I am investigating topology changes in two-dimensional target spaces. I am also interested in Seiberg-Witten invariants. Although much has been learned, some basic questions remain, and I hope to be able at least to understand the simpler of these questionsStanley Mandelstam-Professor Emeritus Particle Theory

As a lay person watching the debate it is difficult for me to discern the basis of these arguments. But I strive to go past what you think is surface in conduct in science's response, as some may show of themself in a reactionary pose. Should we all be so perfect, that the human condition is not also the example by which we shall progress in science?

Dealing in the Abstract



A sphere with three handles (and three holes), i.e., a genus-3 torus.

Of course the thinking may seem so detached from reality that one asks for some reason with which to believe anything. It required, that the history of this approached dust off models in glass cabinets, that were our early descendants of the museum today.

Sylvester's models lay hidden away for a long time, but recently the Mathematical Institute received a donation to rescue some of them. Four of these were carefully restored by Catherine Kimber of the Ashmolean Museum and now sit in an illuminated glass cabinet in the Institute Common Room.

How many of you know how to work in such abstract spaces, and know that what you are talking about has it's relationships in the physics of today? Or that, what satellites we use in measure of, have some correlation to how one may have seen "UV coordinates supplied by Gauss?"

Visual Abstraction to Equations

Sylvester's models lay hidden away for a long time, but recently the Mathematical Institute received a donation to rescue some of them. Four of these were carefully restored by Catherine Kimber of the Ashmolean Museum and now sit in an illuminated glass cabinet in the Institute Common Room.

Some of you might have noticed the reference to the Ashmolean Museum?


Photo by Graham Challifour. Reproduced from Critchlow, 1979, p. 132.

It seems only the good scientist John Baez had epitomes the construction of the Platonic solids? A revision then, of the "time line of history" and the correction he himself had to make? Let's not be to arrogant to know that once we understand more and look at "the anomalies" it forces us to revise our assessments.

The Art form

I relayed this image and quote below on Clifford's site to encourage the thinking of young people into an art form that is truly amazing to me. Yes I get excited about it after having learnt of Gauss and Reimann's exceptional abilities to move into the non euclidean world.

Some think me a crackpot here? If you did not follow the history then how would you know to also include the "physics of approach," as well? Also, some might ask what use "this ability to see the visual abstraction" and I think this art form is in a way destined, to what was kept in glass cabinets and such, even while the glass cabinet in analogy is held in the brain/space of them) who have developed such artistic abilities.

It's as if you move past the layers of the evolution of the human being(brain casings) and it evolution and the field that surrounds them. Having accomplished the intellect( your equations and such), has now moved into the world of imagery. Closet to this is the emotive field which circumvents our perspective on the greater potential of the world in the amazing thought forms of imagery. This move outward, varies for each of us from time to time. Some who are focused in which ever area can move beyond them. This paragraph just written is what would be considered crackpot(I dislike that word)because of the long years of research I had gone through to arrive at this point.

Of course, those views above are different.

Mapping



Is it illusionary or delusional, and having looked at the Clebsch's Diagonal Surface below, how is it that "abstraction" written?

The enthusiasm that characterized such collections was captured by Francis Bacon [1, p. 247], who ironically advised "learned gentlemen" of the era to assemble within "a small compass a model of the universal made private", building

... a goodly, huge cabinet, wherein whatsoever the hand of man by exquisite art or engine has made rare in stuff, form or motion; whatsoever singularity, chance, and the shuffle of things hath produced; whatsoever Nature has wrought in things that want life and may be kept; shall be sorted and included.

There is no doubt that the long road to understanding science is the prerequisite to mapping the images from an equation's signs and symbols. While not sitting in the classroom of the teachers it was necessary to try and move into the fifth dimensional referencing of our computer screen to see what is being extolled here not just in image development, but of what the physics is doing in relation.

In 1849 already, the British mathematicians Salmon ([Sal49]) and Cayley ([Cay49]) published the results of their correspondence on the number of straight lines on a smooth cubic surface. In a letter, Cayley had told Salmon, that their could only exist a finite number - and Salmon answered, that the number should be exactly 27

So of course to be the historical journey was established like most things, Mandelstam current and what is happening there as an interlude, as well as helping to establish some understanding of the abstractions that had been developed.



But yes, before moving to current day imagery and abstraction, I had to understand how these developments were being tackled in today's theoretical sciences.

Beyond the Dance of the Sun

When we first start facing truth, the process may be frightening, and many people run back to their old lives. But if you continue to seek truth, you will eventually be able to handle it better. In fact, you want more! It's true that many people around you now may think you are weird or even a danger to society, but you don't care. Once you've tasted the truth, you won't ever want to go back to being ignorant!

If we concretize thngs and leave no room, then other theories seem like a waste of time compared to our views? Is their no room, to see what perfection the sun has for us in it's rays?


SOHO is a project of international cooperation between ESA and NASA

A lot of times it is much easier to accept the cosmological review of the universe in such a grand scale why would we think we need something more then what is already here? What has the subject of helioseismology to do with the way Wayne Hu may look at his universe?

One of the lessons I learnt as I tried to understand how they tied together the cosmological and quantum world, was to understand that relativity only spoke to that cosmo at large, and that to think anythng more, we would have to bring quantum perspectve in line with it.

We talked lots about micro perspective and particle creation and understood that the beginning of the universe is tied directly to how we micro perceptively deal with it's origins?

You may look at the sun and then realize the dynamical nature such quantum perception reveals as this process continues to unfold for us, as long as the energy is there to support it?

Now that you have shifted your views to the "nature of the dance," I had some choreographies for you to consider.

A Microperspective of the Cosmological world.

A giddy craze was sweeping across Europe at the turn of the 17th century. The wealthy and the well-connected were hoarding things—strange things—into obsessive personal collections. Starfish, forked carrots, monkey teeth, alligator skins, phosphorescent minerals, Indian canoes, and unicorn tails were acquired eagerly and indiscriminately. Associations among these objects, if they were made at all, often reflected a collector's personal vision of an underlying natural "order". Critical taxonomy was rarely in evidence.

I'll just point towards Greg Egans animations in this article.

What are Holonomy figures

While you are seeing these dynamics in context of cosmological reviews would you discard images of the quantum world?), how is abstractness(not real?). Holding many others in thoughts about geometrical propensities from a historical course in projective geometries?

Withn context of a complete revolution, the noting of the solar body and polarity shifts are quite natural, yet, how would you not think of these geometrical dynamcis as how we might look at the "B field" and Cayley shapes?

Just something to add to your thoughts as you concretize your views about let's say, quasars.



I like the Latin name of Sun(Sol). Plato's use of "the sun" in the analogy of the Cave?

And now, I said, let me show in a figure how far our nature is enlightened or unenlightened: --Behold! human beings living in a underground den, which has a mouth open towards the light and reaching all along the den; here they have been from their childhood, and have their legs and necks chained so that they cannot move, and can only see before them, being prevented by the chains from turning round their heads. Above and behind them a fire is blazing at a distance, and between the fire and the prisoners there is a raised way; and you will see, if you look, a low wall built along the way, like the screen which marionette players have in front of them, over which they show the puppets.

Holding a "ideal or image" in mind "as to perfection," can be a guiding light in terms of what possibly "enlightenment" may do for society? What any one moment might do in our realization of what "truly rings the basic core of our understanding" about what we thought long and hard about.:)

A "aha" moment perhaps? A "greater depth of seeing" beyond the "shadows on the walls."

Sylvester's Surfaces

Figure 2. Clebsch's Diagonal Surface: Wonderful.

We are told that "mathematics is that study which knows nothing of observation..." I think no statement could have been more opposite to the undoubted facts of the case; that mathematical analysis is constantly invoking the aid of new principles, new ideas and new methods, not capable of being defined by any form of words, but springing direct from the inherent powers and activity of the human mind, and from continually renewed introspection of that inner world of thought of which the phenomena are as varied and require as close attention to discern as those of the outer physical world, ...that it is unceasingly calling forth the faculties of observation and comparison, that one of its principal weapons is induction, that it has frequent recourse to experimental trial and verification, and that it affords a boundless scope for the exercise of the highest efforts of imagination and invention. ...Were it not unbecoming to dilate on one's personal experience, I could tell a story of almost romantic interest about my own latest researches in a field where Geometry, Algebra, and the Theory of Numbers melt in a surprising manner into one another.

I had been looking for the link written by Nigel Hitchin, as this work was important to me, in how Dynkin drawings were demonstrated. Although I have yet to study these, I wanted to find this link and infomration about James Sylvester, because of the way we might see in higher dimensional worlds.His model seem important to me from this perspective.

Sylvester's models lay hidden away for a long time, but recently the Mathematical Institute received a donation to rescue some of them. Four of these were carefully restored by Catherine Kimber of the Ashmolean Museum and now sit in an illuminated glass cabinet in the Institute Common Room.

The reason for this post is th ework dfirst demonstrated by Lubos Motl and th etalk he linked by Nigel Hitchin. The B-field, which seems to no longer exist, or maybe I am not seeing it in his posts archived?

In 1849 already, the British mathematicians Salmon ([Sal49]) and Cayley ([Cay49]) published the results of their correspondence on the number of straight lines on a smooth cubic surface. In a letter, Cayley had told Salmon, that their could only exist a finite number - and Salmon answered, that the number should be exactly 27

.

There had to be a simplification of this process, so in gathering information I hope to complete this, and gain in understanding.

James Joseph Sylvester (September 3, 1814 - March 15, 1897) was an English mathematician and lawyer.

Now as to the reason why this is important comes from the context of geometrical forms, that has intrigued me and held mathematicians minds. Sometimes it is not just the model that is being spoken too, but something about the natural world that needs some way in which to be explained. Again, I have no teachers, so I hope to lead into this in a most appropriate way, and hopefully the likes of those involved, in matrix beginnings, have followed the same process?

The 'Cubics With Double Points' Gallery




f(x,y,z) = x2+y2+z2-42 = 0,

i.e. the set of all complex x,y,z satisfying the equation. What happends at the complex point (x,i*y,i*z) for some real (x,y,z)?

f(i*x,y,z) = x2+(i*y)2+(i*z)2-42
= x2+i2*y2+i2*z2-42
= x2-y2-z2-42.

Has it become possible that you have become lost in this complex scenario? Well what keeps me sane is the fact that this issue(complex surfaces) needs to be sought after in terms of real images in the natural world. Now, I had said, the B-field, and what this is, is the reference to the magnetic field. How we would look at it in it's diverse lines? Since on the surface, in a flat world, this would be very hard to make sense of, when moved to the three coordinates, these have now become six?

fancier way of saying that is that in general, it's okay to model the space around us using the Euclidean metric. But the Euclidean model stops working when gravity becomes strong, as we'll see later.

Now what has happened below, is that what happens in quark to quark distances, somehow in my mind is translated to the values I see, as if in the metric world and moved to recognition of Gaussian curves and such, to decribe this unique perspective of the dynamics of Riemann, lead through geometrical comprehension ad expression. No less then the joining of gravity to Maxwells world.

Like the magnetic field we know, the lines of force represent a dynamcial image, and so too, how we might see this higher dimensional world. Again I don't remember how I got here, so I am trying hard to pave this road to comprehension.



"Of course, if this third dimension were infinite in size, as it is in our world, then the flatlanders would see a 1/r2 force law between the charges rather than the 1/r law that they would predict for electromagnetism confined to a plane. If, on the other hand, the extra third spatial dimension is of finite size, say a circle of radius R, then for distances greater than R the flux lines are unable to spread out any more in the third dimension and the force law tends asymptotically to what a flatlander physicist would expect: 1/r.

However, the initial spreading of the flux lines into the third dimension does have a significant effect: the force appears weaker to a flatlander than is fundamentally the case, just as gravity appears weak to us.

Turning back to gravity, the extra-dimensions model stems from theoretical research into (mem)brane theories, the multidimensional successors to string theories (April 1999 p13). One remarkable property of these models is that they show that it is quite natural and consistent for electromagnetism, the weak force and the inter-quark force to be confined to a brane while gravity acts in a larger number of spatial dimensions."

Now here to again, we are exercising our brane function(I mean brain)in order to move analogies to instill views of the higher dimensional world. The missing energy had to go somewhere and I am looking for it?:) So ideas like "hitting metal sheets with a hammer", or "billiards balls colliding", and more appropriately so, reveal sound as a manifestation of better things to come in our visions?

See:

Unity of Mathematics