Space and Time: Einstein and Beyond

You know it's amazing sometimes when the question in mind being put out there is not really answered right away, yet, it is strange that you ask and sure enough the right paper seems to find you.

Plato said:Can this difference be as simple as, “a determination between “being discrete, and implying continuity“?



Being stuck on the differences between String/M-theory and Loop Quantum Gravity, it was nice to find this paper and help bring me up to speed.


Prof. Abhay Ashtekar

Quantum geometry is a precise,mathematical theory in which the primary objects-the fundamentals excitations of geometry-are one dimensional. Just as a piece of cloth appears to be smooth, two dimensional continuum although it obviously is woven by one dimensional threads, the Spacetime of General Relativity appears as a four-dimensional continuum although it is in fact, a coherent superposition of these one-dimensional excitations.

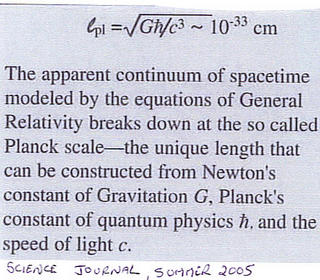
Length Scales

One does not forget the uncertainty at this point, or the relationship of crackpotiness for not mentioning where and how this uncertainty comes into the picture. One must remember I am learning, and the most elegant thing to me so far has been General Relativity and I lay no claims to a theory or a idea, but relationships that pop into mind, that might not always be right. I have certainly come to some conclusion with the way I see tihs dynamcial nature of a quantum world, that hosts all this uncertainty.

If string theory is a theory of quantum gravity, then this minimum length scale should be at least the size of the Planck length,

T-duality is something unique to string physics. It's something point particles cannot do, because they don't have winding modes. If string theory is a correct theory of Nature, then this implies that on some deep level, the separation between large vs. small distance scales in physics is not a fixed separation but a fluid one, dependent upon the type of probe we use to measure distance, and how we count the states of the probe.
This sounds like it goes against all traditional physics, but this is indeed a reasonable outcome for a quantum theory of gravity, because gravity comes from the metric tensor field that tells us the distances between events in spacetime.

Both positons needed to address the "length scale" so this was not to hard a subject to look into once it became apparent, the avenues of discreteness would come into play, as well as continuity. The article linked help with these clarifications.