The Cosmic Triangle

This link was added to paper by Steinhardt as ole picture was used for perspective had been updated, so I gave updated link to Steinhardt, as well as his paper.



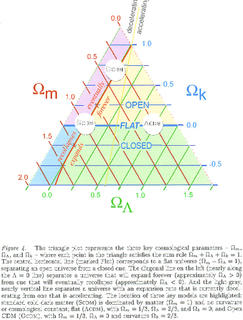
The Cosmic Triangle and the State of the Universe

Moon Phases

I was looking for a moon phase site for a reason and I'll let you know why in a minute here. If you have ever felt the inadequacy of being able to answer such simple questions, I quickly realize how such simple points forced me to go back and understand exactly what the answer must be. This has a way of waking one up to the wonder of all that can be read. All that one might have gained from such research, to realize, how much more there will always be to learn.



My wife, my grandkid's Granmma and I, were making a memory last night before they leave this morning to go home. Now I should say, the night previous to this, our telescopes were set up, for my grandson, from earlier that day had told me what he wanted to spend his allowance on. So we made that trip to do his purchase.

That night we waited for the night sky to come. Being so young, he became tired quickly, and soon fell asleep. There in the north-north east the moon arose, and with it, it''s phase from the 23 of August. Waking our grandson as gently as we could, we asked if he wanted to see the moon. Sleep was stronger then his wonder, I'm afraid.

This is why last night, and with the help of my good wife, we again set up for the moon to arise. This is of course when all the questions about earth, the sun, the moon and so many bombarded Grandpa's intellect for such simple answers. "Why does the earth spin, Grandpa." The quick realization comes for responsible answers. Where shall I find these all these trusty answers?

So as the moon started to rise on the horizon, our kitchen being dark, here shone this computer screen set up for every question, as we punched up google for all these wonders of wonders. "Why do we see the moon, Grandpa?" "Why does the moon stay so close to the earth Grandpa?" It is aversion of, "are we there yet" that Granma quickly saids, "listen Grandpa to what they are saying."

As I sit this morning, it is not to strange that many will sit as grandparents for the generation much younger. Grandparents /Teachers, who will watch education move these younger minds to ask so many questions, and the better educated they become the more difficult these answers.

So I rushed quickly this morning to write about my grandchildren, for it was a wonderful sight to see each of them gaze upon the moons surface. Gain a little knowledge about how long it would take us to get there. How much the moon weights, and on and on.

I too also realized, that as a child of the wonder of it all, that I will also need a grandparent to answer such simple questions. So it would be with such patience and clarity, that such answers can be given in the hopes that as chldren, we will be quietly nudged from those ahead of us, in age and education, that such patience shall rule the day, as it must for the quiet and absorbed mind of a child that askes.

This I learnt last night, and how wonderful to see such participaton and questions. Such "tolerance" from those who blog. To set aside and be examples, for those in general, will reach out to try and touch that understanding they have.

View on the Expansion of the Universe

Georgi Dvali:The cosmic acceleration of the universe indicates that the laws of General Relativity get modified not only at very short but also at very large distances, Dvali says. It is this modification, and not dark energy, that is responsible for the accelerated expansion of the universe.

What Do Cosmologists want from String Theory?

More Views on the Landscape

How would such a "landscape" bring string theory into better comprehension? The
Most perfect fluid in face of strong coupling?

Yet at the other end, there is no deviation?

Eric Adelberger on Aug 12th, 2005 at 2:37 pm It is true that we are seeing an anomaly at shorter length scales but we have to show first that the anomaly is not some experimental artifact. Then, if it holds up, we have to check if the anomaly is due to new fundamental physics or to some subtle electromagnetic effect that penetrates our conducting shield. We are now checking

Lubos being on top of it, reveals new paper.

New Constraints on Yukawa-Type Deviations from Newtonian Gravity at 20 Microns

Recent theories of physics beyond the Standard Model have predicted deviations from Newtonian gravity at short distances. In order to test these theories, we have a built an apparatus that can measure attonewton-scale forces between gold masses separated by distances on the order of 25 microns. A micromachined silicon cantilever was used as the force sensor, and its displacement was measured with a fiber interferometer. We have used our measurements to set bounds on the magnitude alpha and length scale lambda of Yukawa-type deviations from Newtonian gravity; our results presented here yield the best experimental limit in the range of lambda=6--20 microns.

Don't cater to Intelligent Design debate Please?

I would say attitudes from respectable scientists had more to do it with then any intelligent design debate. Any repeated pattern of news, associated. This debate was fuelled.

While respected the stand taken against any position on "intelligent design" it would have been outright diagreement with the religious connotation for sure, then argue the basis of science assign to a differing group opinion. I stayed away from the source of this debate arising, as I watched Sean, Peter Woit and others villify and characterise(news jumped on this), a respective theoretical view, based on such association.

This hurt propsective views on recogniton of theoretical position.

The debate had to be rested in science, and any distinction of religion dropped from the conversation. Only the reason why such rejection, and such slogans pasted on other views contained. Lee Smolins attempt to draw other so science, and Lubos's attempted to clarify current scientific data. Argue then, Georgi Dvali's moon measure?

What Lies Beneath

The Bottom up approach?

R.B.Laughlin:

The paper by Senthil et al. [9] is an attempt to address this issue mathematically. It deals specifically with a suspicion many of us have had that quark confinement, one of the most cherished features of the standard model, may be a collective effect that emerges at a phase transition and thus not fundamental at all. The paper is complicated, an unfortunate side effect of the difficulty of the task, for it is not generally possible to deduce emergent phenomena from first principles. The best one can do is postulate them and then demonstrate plausibility by showing that small corrections get smaller as the measurement scale increases. Such convoluted arguments are ripe with opportunities for mistakes, regardless of how careful the authors have been, so the test of emergent universality that counts is always experimental. This, in turn, forces the theory to address not quark confinement itself but an allegory of it one might hope to test in a table-top experiment. The logic is maddeningly indirect, but unfortunately the only approach that is legitimately scientific.

Fig. 1. In quantum chromodynamics, a confining flux tube forms between distant static charges. This leads to quark confinement - the potential energy between (in this case) a quark and an antiquark increases linearly with the distance between them.



In the Q<->Q measure, the understanding of this distance in the metric was understandable?


Now this is March of 2000.


What Lies Beneath?



Still as a layman, such general talks need better clarification? If you set the stage from planck length, then how indeed does LQG arise here?

Here's another view.

Witten:One thing I can tell you, though, is that most string theorist's suspect that spacetime is a emergent Phenomena in the language of condensed matter physics.

http://online.kitp.ucsb.edu/online/kitp25/witten/oh/10.html

Robert Laughlin:The true origin of these rules is the tendancy of natural systems to organize themselves according to collective principles. Many phenomena in nature are like pointillist paintings. Observing the fine details yields nothing but meaningless fact. To cor rectly understand the painting one must step back and view it as a whole. In this situation a huge number of imperfect details can add up to larger entities of great perfection. We call this effect in the physical world emergence.

http://large.stanford.edu/rbl/lectures/index.htm

Observatories

What is Sun-Earth Day?

As I was reading Cliffords newest entry on Cosmic Variance site, his trip reminded me of the hike we took to see the Big Horn Medicine Wheel.

Now what is interesting about this is I am not one who has had much association with such places of observatories, but far up to the left of this wheel is one that seems very out of place.

Ironic in the sense that ole history about these "Medicine Wheels" could have brought such historical perspective to the science. Look at the universe, from a place where ancient artifacts gathered.

At the center of the wheel there is a raised central cairn, and several others on the periphery of the wheel. These have been alleged to have astronomical alignments. Astronomer John Eddy suggested that a line drawn between the central cairn and an outlying cairn at the Bighorn Medicine Wheel pointed to within 1/3 of a degree of the rising point of the sun at the summer solstice. The actual astronomical purpose of the design of these wheels remains controversial. The design may also have assisted in the performance of specific rituals and ceremonies that have been lost to us. The 28 spokes could indicate the lunar month, or the length of the female menstrual cycle.

But I am not going to join the speculative feature of this wonderment, but to bring forward the understanding that mountains that may look the same, may have other reasons like it does for Clifford and his views of home. That for him to look, and have something nagged his "observatory mind" would have been as simple as "cheez, it looks like home."

Well from a more suttle place, I bring forward the understanding that our perspective about cosmology, our understanding of the uniqueness of Omega? The implications of General Relativity, and how Alexanders Firedmann's eqaution is part and parcel of the understandng of a geometry. That helps lead the mind into the ability to see dynamicals of this universe. How would your obervation have changed with such paradigmal changes. In Toposense, is speak to that as well as relate that General Realtivity had it's saem consequence. Ask Sean about that one?

Now we engage the spacetime fabric. This dynamcial abiltiy would not be seen before without this geometrical prospensity. So having been taken over by paradigmal change, the visionistic approach is one based on geometrical design, where the uniqueness of such correlations in the views of where nature resides. Brings one closer to the very spots we call "home". This is a real place for clifford, and yet without inducing such mysticism, this is also a place I draw from.

Now you say this guy is nuts. But imagine the science that leads one to see such topolgical realizations would take cosmological priciples about this epxanding universe and find that in a Genus example of the spherical WMAP of creation, there are abilities of this universe to become, well, lets say like images of marbles on rubber sheets, and how did such isolated cases exist within the greater potential of this universe to unfold and one is lead to portions of objective collapses that help to bring a greater dynamcial view about this same cosmos.

What makes this different is I relate topo-sense as a real part of paradigmal change.

The theory of relativity predicts that, as it orbits the Sun, Mercury does not exactly retrace the same path each time, but rather swings around over time. We say therefore that the perihelion -- the point on its orbit when Mercury is closest to the Sun -- advances.

I encourage such changes when we learn( or are really a result of such learning). Who could not get this sense from, Mercuries Daisey, or Hulse and Taylor Binary star rotations that release "gravitation waves" that give us information about how close they are becoming. What's it's predictve date about coming together?

While I relate Mecuries orbital patterns, such sense is not limited to here. Other idealizations as well, that we might wonder indeed how vast this landcape idea, when you consider the >Lagrange points?:)

Space and Time: Einstein and Beyond

You know it's amazing sometimes when the question in mind being put out there is not really answered right away, yet, it is strange that you ask and sure enough the right paper seems to find you.

Plato said:Can this difference be as simple as, “a determination between “being discrete, and implying continuity“?



Being stuck on the differences between String/M-theory and Loop Quantum Gravity, it was nice to find this paper and help bring me up to speed.


Prof. Abhay Ashtekar

Quantum geometry is a precise,mathematical theory in which the primary objects-the fundamentals excitations of geometry-are one dimensional. Just as a piece of cloth appears to be smooth, two dimensional continuum although it obviously is woven by one dimensional threads, the Spacetime of General Relativity appears as a four-dimensional continuum although it is in fact, a coherent superposition of these one-dimensional excitations.

Length Scales

One does not forget the uncertainty at this point, or the relationship of crackpotiness for not mentioning where and how this uncertainty comes into the picture. One must remember I am learning, and the most elegant thing to me so far has been General Relativity and I lay no claims to a theory or a idea, but relationships that pop into mind, that might not always be right. I have certainly come to some conclusion with the way I see tihs dynamcial nature of a quantum world, that hosts all this uncertainty.

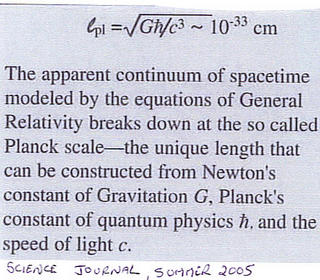
If string theory is a theory of quantum gravity, then this minimum length scale should be at least the size of the Planck length,

T-duality is something unique to string physics. It's something point particles cannot do, because they don't have winding modes. If string theory is a correct theory of Nature, then this implies that on some deep level, the separation between large vs. small distance scales in physics is not a fixed separation but a fluid one, dependent upon the type of probe we use to measure distance, and how we count the states of the probe.
This sounds like it goes against all traditional physics, but this is indeed a reasonable outcome for a quantum theory of gravity, because gravity comes from the metric tensor field that tells us the distances between events in spacetime.

Both positons needed to address the "length scale" so this was not to hard a subject to look into once it became apparent, the avenues of discreteness would come into play, as well as continuity. The article linked help with these clarifications.

Big Horn Medicine Wheel

I'll put a picture here from my trip(it is taken from the board located at the site). It's the Bighorn Medicine Wheel located by Sheridan Wyoming. It is about 9642 feet up and on Medicine Mountain. The walk was about a mile and half in and was about a 100 degrees that day.

If conceived as a series of ever-wider experiential contexts, nested one within the other like a set of Chinese boxes, consciousness can be thought of as wrapping back around on itself in such a way that the outermost 'context' is indistinguishable from the innermost 'content' - a structure for which we coined the term 'liminocentric'.

So these artifacts of culture and methods of model intepretation, can be one of many that finds interesting features, as a expansitory universe? At the same time such model enhances the internal developement. No wonder the Klein bottle attracts the eye, or a mobius strip. And let's not be sheepish here, the Taoist symbol? IMagine indeed one could suffer the wrath of Gellman and look out Tony Smith you might be sidelined because of these yin and yang symbolizations and the Eightfold way?

Culture changes perspective as much as new models that help us see things in different ways.

The familiar extended dimensions, therefore, may very well also be in the shape of circles and hence subject to the R and 1/R physical identification of string theory. To put some rough numbers in, if the familiar dimensions are circular then their radii must be about as large as 15 billion light-years, which is about ten trillion trillion trillion trillion trillion (R= 1061) times the Planck length, and growing as the universe explands. If string theory is right, this is physically identical to the familiar dimensions being circular with incredibly tiny radii of about 1/R=1/1061=10-61 times the Planck length! There are our well-known familiar dimensions in an alternate description provided by string theory. [Greene's emphasis]. In fact, in the reciprocal language, these tiny circles are getting ever smaller as time goes by, since as R grows, 1/R shrinks. Now we seem to have really gone off the deep end. How can this possibly be true? How can a six-foot tall human being 'fit' inside such an unbelievably microscopic universe? How can a speck of a universe be physically identical to the great expanse we view in the heavens above? (Greene, The Elegant Universe, pages 248-249).

What relevance would Greene's statement have in relation, and the draw back of meaning other then the support of physics, makes my subjectives perceptions questionable?

But from the understanding of the expansion of the cosmos, what model is hidden in our perceptions, that we would seek to make the journey of humanity whole? By seeing and understanding, the beginning and the end of this universe, is a suttle change from one phase state change, to the next? From Planck Epoch to Grand Unification, and how suttle(high) the energy that makes its way in our world to see it is measured and in wonderous mode, call it the Oh my God Particle?

So then, the history of that universe is with us now. Where the possibility exists that such microstate blackholes would have the opportunities to indicate what shall begin and from where?

So what are we to think of this dimensional perspective that would describe for us this anomalie of Eric Adelberger on Aug 12th, 2005 at 2:37 pm, experimentally is being done for the measure of those extra dimensions?

Unexpected High energies of Cosmic Rays

Plato said:

I am thinking about Lee Smolin’s history here in terms of discrete measures ( I am developing a perspective here in relation that will be complied later) How this effected the the way Lee may have viewed the background. I don’t want to speak for Lee Smolin, but I would like to make it simple.:)

Can this difference be as simple as, “a determination between “being discrete, and implying continuity“?

Where strings implying only tree features, while the approach to glast, as a “new view” supported by "Doubly Special Relativity", that Rovelli and Lee produced? This basis and history is what I am compiling.

One can ask any question and have it loaded, with lots of information. But just trying to bring something to simple clarity, even in conceptual framesworks is not always easy, if you don' ask the question?

Greisen-Zatsepin-Kuzmin limit

The Greisen-Zatsepin-Kuzmin limit (GZK limit) is a theoretical upper limit on the energy of cosmic rays from distant sources.

This limit was computed in 1966 by Kenneth Greisen, Vadem Kuzmin and Georgi Zatsepin, based on interactions predicted between the cosmic ray and the photons of the cosmic microwave background radiation. They predicted that cosmic rays with energies over the threshold energy of 5×1019 eV would interact with CMB photons to produce pions. This would continue until their energy fell below the pion production threshold. Therefore, extragalactic cosmic rays with energies greater than this threshold energy should never be observed on Earth.

Unsolved problems in physics: Why is it that some cosmic rays appear to possess energies that are theoretically too high, given that there are no possible near Earth sources, and that rays from distant sources should have been absorbed by the cosmic microwave background radiation?A number of observations have been made by the AGASA experiment that appear to show cosmic rays from distant sources with energies above this limit (whimsically dubbed Oh-My-God particles). The observed existence of these particles is the so-called GZK paradox or cosmic ray paradox.

Anyway, this was brought up and the questioned asked, because I did understand something that even if it was based on theoretical definitions might have been ones that were different from another, and brought the scorn of high energy physicists to wonder, where such principles had been raised in terms of quantum gravity?

So lookng at Lee's position and it becomes clear when one does the research on cosmological scenarios, that no wonder you want the string theorist on side, in this debate.

John Ellis is a fine educator when it come to laying the simple view to avenues related to both High energy physics and the relation in Pierre Auger determination.

Imagine microstate blackholes, and I wonder what "this trigger is" that would make life so much easier if we could determine the background,versus non background debate in terms of these experimental positions?



So strings and Loop quantum are face to face here in our informational predictions, about outcomes of the background versus the non-background, and getting to the source of this debate, from a physics interpretation and a expeirmental one, has always been the quest I think, and one supported by Lee Smolin.

So what rationality might have issue from the basis of that theoretcial position, and like I said before, it seems what pubic relations the top scientists have with the public, is to lay the foundation at the front door in a simple a way as possible from the blogosphere. What other way is so simple and direct tot he public that such distance could now been narrow to someone like Clifford in Cosmic INvariance, speaking to this very subject. Any the link below this sets the tune, and here th econtinuation of th equestion I had there that has not been repsonded too, becuase of the layman underdevleoped view of where top theoretorcians reside.

I'll give it a stab anyway. There seems to be a certain romance I have with the subject, that does not require money from any avenue, and such grants, far from the layman's view that doing this for fun, has been most rewarding becuase it brought me to see in different ways in the bulk, that others in simple life care not, and walk their way.

Plato said:

Seeking clarity in relation to experimental propositions of Glast 2006 and how it shall support one’s position over another? Will it?

High energy relevance had to meet each other in a way that cosmologically had something to do with high energy perceptions in relation to the trigger? Link on name.

The “beginning”, as first principles? Robert Laughlin saids no to “first principles”?:)

Since it is hard to put a link within a link, I thought I better put link on name here as well.

I really hate quoting myself, but alas the move is to continue regardless, so onto, "Gubser and structure information."

Lubos Motl said:

Steve Gubser (from Princeton) has just gave an interesting talk at the joint seminar in which he tried to convince us that structure formation (the process in which the early clumps of matter and the first galaxies were born) is a very interesting topic in cosmology, even for string theorists, in which some signs of new physics may be found if one tries to reproduce the observations.

How indeed would one see gluonic perception at this level bringing us ever closer to views on the window of the universe, and such leaidng indicators has to bring some noton to what started in the beginning? Non?

Explanation on the Landscape

Photograph by Clifford Johnson

While on Cosmic Invariance, Clifford brings a much needed attempt at explanation on how we view the landscape. I'll have to spend sometime going over this becuase it is a critical position and difference between two facets of thinking within the scientific community. Susskind and Lee Smolin have lead this discussion repeatedly before, and I find this continued effort, a nice way to continue to peer into.

Some response helps too, and indicates our attention to the reasons why such positions are adopted. I find this very important in understanding why these respective positions, are taken and what possibly might issue from the stated position.

Lee Smolin on Aug 15th, 2005 at 9:01 am :

Method A: ASSUME 1) that there is a real non-perturbative theory behind all the approximate calculations and 2) that it is relevant for nature. Then interpret various results, having to do with dualities, the landscape etc given these assumptions.

Method B: Look for evidence that the two assumptions of method A are true.

One evaluates results very differently, depending on whether one uses method A or method B. There is nothing wrong with using Method A from time to time, so long as the assumptions are made explicit, and the risks that are thereby taken on explicitly acknowledged. One can learn things that will turn out be true about the theory, if 1) is true, or about nature, if 2) is true. But one cannot do science only or even mostly by Method A, no matter how promising an idea may seem. What I find disturbing in your essay, and in many conversations with string theorists is that they reason by Method A but they do not state explicitly their assumptions. This puts me often in the uncomfortable situation, when discussing with a string theorist, of having to add, “but there is one more possibility, the theory might be wrong.”

So Jacque Distler adds his views and I heard he walked out on the conference? So am I to take it that this very topic tries his impatience, that he might have seen bias raise it's ugly head, or that holding a position like Peter Woit's put them the odd man out? I think Lee is doing a fine Job of trying to keep cohesion amongst the scientists that we do not have to worry, about such antics, as they eventually come around to accept the debate?:)

Jacques Distler on Aug 15th, 2005 at 10:52 am

Lee was, most recently, at Strings 2005, and he does hang out with his stringy colleagues at Perimeter.

I don’t know whether that counts for you, but it does for me.

The Layman's view

As stated before in how Clifford presents his perspective I immediately noticed a corresponding image in my mind in terms of hypherysics.



Now you have to forgive my laymen perspective because when they start talking about the landscape, I tend to see this completed image in my mind, much as I have relayed it here(Are Scientists Currently Censoring Debate on Global Warming). Although it seeks to detail the environment as a relative view on such perspective as landscape, I thought I would see how Cliiford's view and the resulting talks might have been understood from my own perspective.

Unfortunately I do not have the guidance other then what I can intuitive garner in my continue development, so I hope I do other justice and do not degrade this topic in any way.

The ground state of a three-dimensional box of dimension L can be obtained by setting n=1 for all three dimensions, giving an energy three times the ground state energy of the one-dimensional box. The ground state for the three-dimensional box would be

So when one looks at these images of the landscape what is being said here, I tried to garner a overall perspective as I did in the "censoring debate on Global warming".



I give a direct link to the picture that had been presented early on in my research, because I tended to see this split very early on as a positional one worth taking note. But somewhere along the line my thinking changed as I saw the vast differences and capabilities of the bulk possibilities in terms of graviton scattering and condensing feature.

So transferring this thinking to global differences help me to continue to see how Clifford or string theory approach to landscape development might be seen. I rest easy that there are those better qualified, but this has not limited what I have now been able to see. As others will see in the landscape interpretation.